Concrete in nature is poor in tension, thus steel reinforcement is provided to improve the tension. The combination of steel and concrete makes the ideal construction materials for the structure members. The bond strength of the steel and concrete is defined by development length.
What is Development Length?
A development length can be defined as the length of the reinforcement bar needed to be embedded or projected into the column to establish the desired bond strength between the concrete and steel (or any other two types of material).
Importance of Development Length
The importance of providing development length at the junction of the column footing or column beam is crucial to ensure sufficient protection for the bar surface. The following are the primary reasons behind providing a development length.
- Ensuring the bar’s stability within the concrete and preventing any displacement.
- Preventing any potential damage caused by bar sliding under extreme loads.
- Facilitating the transfer of pressures generated in one area to neighbouring sections by incorporating additional bar length, as specified in the production length at the column.
- The specified length at the beam-column junction allows for the effective transfer of stresses through the steel bond, facilitating the transfer of forces.
Derivation of Development Length
A steel bar is embedded in concrete as shown in Figure 1. The bar carries a tensile force T equal to Ast. σst. The bond force developed in the steel bar is equal to the tensile force and acts in the opposite direction. The steel bar is in equilibrium. Therefore,
\( \tau_{bd}L_d\pi\phi=\left[\left(\frac\pi4\right).\phi^2\right]\sigma_{st} \) …eq1
\( \sigma_{st=0.87f_y} \) …eq2
\( L_d=\frac{\left(0.87f_y\right).\phi}{4.\tau_{bd}} \) …eq3
Therefore, the development length Ld is given by
\( L_d=\frac{\phi.\sigma_s}{4.\tau_{bd}} \) …eq4
\( L_d \)= Development Length
ø = Diameter of steel bar
σs = Stress of steel bar
\( \style{font-family:’Times New Roman’}{\style{font-size:18px}{\tau_{bd}}} \) = Design bond Stress
Design bond stress for plain steel bar and HYSD steel bar in limit state is given in Table 1 and Table 2 according to IS 415:2000.
| Grade of concrete | M15 | M20 | M25 | M30 | M35 | M40 and above |
|---|---|---|---|---|---|---|
| Design bond stress, \( \style{font-family:’Times New Roman’}{\style{font-size:18px}{\tau_{bd}}} \), N/mm2 | 1.0 | 1.2 | 1.4 | 1.5 | 1.7 | 1.9 |
| Grade of concrete | M15 | M20 | M25 | M30 | M35 | M40 and above |
|---|---|---|---|---|---|---|
| Design bond stress, \( \style{font-family:’Times New Roman’}{\style{font-size:18px}{\tau_{bd}}} \), N/mm2 | 1.6 | 1.92 | 2.24 | 2.4 | 2.72 | 3.04 |
Also, read: Types of Steel Reinforcement Bars
Calculation of Development Length
For the calculation of Ld, let’s first consider the grade of concrete as M20 grade and steel as Fe415.
σs = 0.87 x 415
= ( ø x 0.87 x 415 ) / ( 4x 1.92 )
Ld = 47.012ø ~ 47ø
Therefore, Ld = 47ø …eq5
The bar of 20 mm diameter is simply the multiplication of 47 and the diameter of the bar.
=47 x 20
=940 mm
Development length for various grades of concrete and Steel Bar
The values of design bond stress in tension and the development length (Ld) for plain mild steel bars and HYSD-steel bars for different grades of concrete as specified in IS: 456–1978 are given in the Table below.
| Grade of Concrete | Plain Mild Steel Bars Fy=250 N/mm2 | Plain Mild Steel Bars Fy=450 N/mm2 | ||
|---|---|---|---|---|
| τbd | Ld | Zu | Ld | |
| M15 | 1.00 | 54.375Ø | 1.60 | 56.414Ø |
| M20 | 1.20 | 45.313Ø | 1.92 | 47.012Ø |
| M25 | 1.40 | 38.839Ø | 2.24 | 40.296Ø |
| M30 | 1.50 | 36.250Ø | 2.40 | 37.185Ø |
| M35 | 1.70 | 31.985Ø | 2.72 | 33.185Ø |
| M40 | 1.90 | 28.618Ø | 3.04 | 29.692Ø |
Factor Influencing Development Length
Several factors affect the development length, and it’s essential to consider these factors for designing structurally sound and safe constructions. Here are some of the key factors:
Concrete Strength (Compressive strength):
Higher concrete strength generally leads to a shorter development length. As the compressive strength increases the development length also decreases as seen in the table above.
Read more on: Compressive Strength Of Concrete
Bar Diameter:
The diameter of the reinforcement bar is also one of the most important factors which influences the development length. From equation 4, it is understood that the development length is directly proportional to the diameter of the bar. Hence, the larger the bond length between the concrete and steel as the diameter bar increases.
Also, read: Compressive Strength Of Concrete
Types of Development Lenght
1. Bond Development Length
Bond development length refers to the distance along the length of a reinforcing bar (rebar) over which the bar provides adequate bond or adhesion to the surrounding concrete. When concrete is subjected to loads, such as tension or compression, the reinforcing bars within it are responsible for carrying these loads. The bond development length is the distance required to ensure that the stresses transferred between the concrete and the reinforcing bar are effectively distributed.
2. Anchorage Length
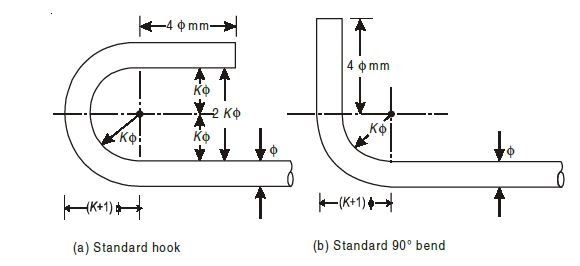
The anchorage length shall be considered while providing the reinforcement bar at the junction and critical section of the structure members such as footing, slabs and staircase. The anchorage length is provided in the tension and compression bar by either a standard hook or bend. The standard hook and bend are provided as shown in figure 2.
Also, read: How To Calculate Unit Weight of Steel Bar
2.1. Anchorage Length of The Tension Bar
The anchorage length is not necessarily provided to the tension bar when HYSD steel is used. Hooks are provided for plain bars in tension. Bends and hooks shall conform to IS 2502.
Bend: – The anchorage value should be set to 4 times the diameter of the bar, for each 45° bend subjected maximum of 16 times the diameter of the bar.
Hook: – The anchorage value of a standard U-type hook shall be equal to 16 times the diameter of the bar.
2.2. Anchorage Length of The Compression Bar
This is the minimum length of the bar provided at the junction of the beam and column to prevent the failure of the joints under certain loading conditions. The anchorage length of the compression bar should be equal to the development length. The total length of anchorage length should include a hook, bent and straight length of the bar if provided.
Lap Splice Length
This is the portion where the main bar gets terminated due to inadequate length and continues with the same properties as the other bar. These bars are joined either by overlapping known as lapping. The length of the lapping is equal to the development length in tension reinforcement bars.
Also, read: What are Structural Steels? | Types | Usage
FAQs
1: What is development length?
A: Development length is the length of the reinforcement bar that is required to be embedded into the concrete to develop the full tensile capacity of the bar.
2. What is the formula to calculate development length?
A: The formula to calculate development length is given by:
\( L_d=\frac{\phi.\sigma_s}{4.\tau_{bd}} \)
where,
Ld = Development Length
ø = Diameter of steel bar
σs = Stress of steel bar
\( \style{font-family:’Times New Roman’}{\style{font-size:18px}{\tau_{bd}}} \) = Design bond Stress
3: What is the minimum development length for the reinforcement bar?
A: The minimum development length of the reinforcement bar is calculated by using the formula 47ø for the fy415 and M20 grades of concrete. Where ø is the diameter of the bar.
4: Why is development length important in reinforced concrete design?
A: Development length is important because it ensures that the full tensile capacity of the reinforcement bar is utilized to resist the applied load. If the development length is insufficient, it can result in premature failure of the concrete element.
5: What factors affect development length?
A: The factors that affect development length include the strength of the concrete, the diameter and grade of the reinforcement bar, the shape and texture of the bar, the degree of confinement, and the presence of any additional reinforcement or prestressing.
References:
- ACI 318-11. (August 2011). Building Code Requirements for Structural Concrete
- Indian Standard. (2000). Code of Practice for Plain and Reinforced Concrete (IS 456:2000) (4th ed.). Bureau of Indian Standard (BIS).
- Indian Standard. (1963). Code of Practice for Bending and Fixing of Bars for Concrete Reinforcement (IS 2502-1963). Bureau of Indian Standard.
- Indian Standard. Code of Practice for Ductile Design and Detailing of Reinforced Concrete Structures Subjected to Seismic Forces (IS 19320- 2016). Bureau of Indian Standard.
- Housing News. (2023). Development length formula: Know meaning and calculation. Housing News. https://housing.com/news/what-is-development-length-in-construction-work/
- Mishra, G. (2018). Development Length of Reinforcement Bars. The Constructor. https://theconstructor.org/structural-engg/development-length-reinforcement-bars/1344/
![]()